How To Find Final Volume In Chemistry

The behavior of gases can be described by several laws based on experimental observations of their properties. The pressure of a given amount of gas is directly proportional to its absolute temperature, provided that the volume does not change (Amontons's law). The volume of a given gas sample is directly proportional to its absolute temperature at constant pressure (Charles's law). The volume of a given amount of gas is inversely proportional to its pressure when temperature is held constant (Boyle's law).

Under the same conditions of temperature and pressure, equal volumes of all gases contain the same number of molecules (Avogadro's law). Eventually, these individual laws were combined into a single equation—the ideal gas law—that relates gas quantities for gases and is quite accurate for low pressures and moderate temperatures. We will consider the key developments in individual relationships , then put them together in the ideal gas law. As with w/v solutions, we weigh out a specific amount of chemical when making a molar solution. Unlike w/v solutions, the amount to weigh depends on the molecular weight (m.w.) of the substance in grams per mole (g/mol). In order to calculate the desired mass of solute you will need to know the formula weight.

Formula weights are usually printed on the label and identified by the abbreviation f.w. Formula weight is the mass of material in grams that contains one mole of substance, and may include inert materials and/or the mass of water molecules in the case of hydrated compounds. For pure compounds the formula weight is the molecular weight of the substance and may be identified as such. In contrast to other gas components, water content in air, or humidity, to a higher degree depends on vaporization and condensation from or into water, which, in turn, mainly depends on temperature. Therefore, when applying more pressure to a gas saturated with water, all components will initially decrease in volume approximately according to the ideal gas law. However, some of the water will condense until returning to almost the same humidity as before, giving the resulting total volume deviating from what the ideal gas law predicted.

Conversely, decreasing temperature would also make some water condense, again making the final volume deviating from predicted by the ideal gas law. Perhaps the easiest way to describe a solution is in terms of weight-in-weight (w/w). The weight of the solute relative to the weight of the final solution is described as a percentage. For example, suppose you have a dye that is soluble in alcohol. Rather than write the instructions, "take 3 grams dye and mix with 97 grams absolute alcohol," you can describe the solutions simply as 3% dye in absolute alcohol. The formula applies to any volume of solution that might be required.

Three grams dye plus 97 grams alcohol will have final weight of 100 grams, so the dye winds up being 3% of the final weight. Note that the final weight is not necessarily equal to the final volume. Aqueous weight-in-weight solutions are the easiest to prepare. Since 1 milliliter of water weighs one gram, we can measure a volume instead of weighing the solvent.

A very common use of w/w formulas is with media for the culture of bacteria. Such media come in granular or powdered form, often contain agar, and often require heat in order to dissolve the components. Microbiological media, especially when they contain agar, are difficult to transfer from one vessel to another without leaving material behind.

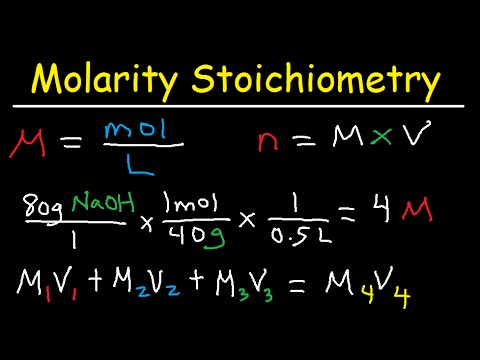
They coat the surfaces of glassware, making quite a mess. Using a w/w formula the media and water can be mixed, heated, then sterilized, all in a single container. Very little material is wasted and there is less of a mess. It is important to note that the molarity is defined as moles of solute per liter of solution, not moles of solute per liter of solvent. This is because when you add a substance, perhaps a salt, to some volume of water, the volume of the resulting solution will be different than the original volume in some unpredictable way.

To get around this problem chemists commonly make up their solutions in volumetric flasks. These are flasks that have a long neck with an etched line indicating the volume. The solute is added to the flask first and then water is added until the solution reaches the mark. The flasks have very good calibration so volumes are commonly known to at least four significant figures. Charles' law is a special case of the ideal gas law in which the pressure of a gas is constant.

Charles' law states that volume is proportional to the absolute temperature of a gas at constant pressure. Doubling the temperature of gas doubles its volume, so long as the pressure and quantity of the gas are unchanged. This relationship between temperature and pressure is observed for any sample of gas confined to a constant volume.

An example of experimental pressure-temperature data is shown for a sample of air under these conditions in Figure 9.11. The volume and temperature are linearly related for 1 mole of methane gas at a constant pressure of 1 atm. If the temperature is in kelvin, volume and temperature are directly proportional. Charles's law states that the volume of a given amount of gas is directly proportional to its temperature on the kelvin scale when the pressure is held constant.

Gases whose properties of P, V, and T are accurately described by the ideal gas law are said to exhibit ideal behavior or to approximate the traits of an ideal gas. An ideal gas is a hypothetical construct that may be used along with kinetic molecular theory to effectively explain the gas laws as will be described in a later module of this chapter. Although all the calculations presented in this module assume ideal behavior, this assumption is only reasonable for gases under conditions of relatively low pressure and high temperature. In the final module of this chapter, a modified gas law will be introduced that accounts for the non-ideal behavior observed for many gases at relatively high pressures and low temperatures.

As you mixed the two liquids, the ethanol and water molecules began to interact with each other, packing together in a different way than in either pure ethanol or pure water. This results in a new density of the solution and thus, a volume that does not equal the sum of the two original volumes. In other words, the volume of solution, the denominator of our volume percent equation, does not equal the volume of solute plus the volume of solvent.

In the case of water and ethanol, their mixture has less volume than the sum of the pure liquids. A disadvantage of describing formulas as w/v (%) is that the description says nothing about the actual concentration of molecules in solution. What if we want equal amounts of two chemicals to be mixed together, so that for each molecule of substance #1 there is a single molecule of substance #2? The same amount in grams will likely not contain the same number of molecules of each substance. For example, you may work with a chemical that can be in one of several forms of hydration. Calcium chloride can be purchased as a dry chemical in anhydrous form, so that what you weigh out is nearly all pure calcium chloride.

On the other hand you may have a stock of dry chemical that is hydrated with seven water molecules per molecule of calcium chloride. The same mass of this chemical will contain fewer molecules of calcium chloride. When we are interested in the actual concentration of molecules of a chemical in solution, it is better to have a universal measurement that works regardless of how the chemical is supplied. As long as the molecular weight is known, we can describe a solution in the form of moles per liter, or simply molar .

1 mole of an ideal gas is compressed isothermally from a volume of 500 cm3against a constant external pressure of 1.216 × 105 Pa. The pressure-volume work involved in the process is 36.5 J. Energy is transferred along with the genetic material and so obeys the first law of thermodynamics. Energy is transferred—not created or destroyed—in the process.

When work is done on a cell or heat transfers energy to a cell, the cell's internal energy increases. When a cell does work or loses heat, its internal energy decreases. In thermodynamics, the volume of a system is an important extensive parameter for describing its thermodynamic state.

The specific volume, an intensive property, is the system's volume per unit of mass. Volume is a function of state and is interdependent with other thermodynamic properties such as pressure and temperature. For example, volume is related to the pressure and temperature of an ideal gas by the ideal gas law.

When we describe a concentration as a percentage without specifying the type of formula, we imply that the solution is to be made using the weight-in-volume (w/v) method. As with w/w, weight-in-volume is a simple type of formula for describing the preparation of a solution of solid material in a liquid solvent. Boyle's law, together with Charles's law and Gay-Lussac's law, are among the fundamental laws which describe the vast majority of thermodynamic processes. We have gathered them all in our thermodynamic processes calculator, where you can choose whichever process you like, and evaluate the outcomes for a real gases.

Calculate the final volume of one mole of an ideal gas initially at 0oC and 1 atm pressure. If it absorbs 2000 cal of heat during reversible isothermal expansion. Human metabolism is the conversion of food into energy given off by heat, work done by the body's cells, and stored fat. Metabolism is an interesting example of the first law of thermodynamics in action. Eating increases the internal energy of the body by adding chemical potential energy; this is an unromantic view of a good burrito. 3 moles of an ideal gas are expanded isothermally from volume of 300 cm3to the volume 2.5 L against a constant external pressure of 1.9 atm at 300 K.

Consider an ideal gas having definite mass be enclosed in a cylinder fitted with weightless, frictionless, tightly fitted, movable piston. Let 'A' be the area of the cross-section of the cylinder. Let the gas expand from volume V1 to V2 against constant external pressure Pext which is exerted on the piston. Due to expansion, the piston moves upward by a distance 'd'. In this process, the system loses energy to the surroundings. Most chemicals tend to absorb water unless they are kept desiccated, that is to some extent they are hydroscopic.

This problem should not be confused with the state of hydration of a substances, which refers to the direct association of water molecules with molecules of the substance through hydorgen bonding. Magnesium chloride is commonly used in biological buffers, and is notoriously hydroscopic. It is usually not practical to worry about water content, since it is so difficult to control.

If precision is critical, then chemicals should be maintained under desiccating conditions or used immediately before they can absorb a significant amount of water. Suppose that someone has already worked out the details, so all that you have to do is read a formula and make a solution. We can usually assume that a solution is to be aqueous unless stated otherwise.

What about the concentration of the substance to be added? Common ways of describing the concentrations of solutions are weight-in-weight, weight-in-volume, volume-in-volume, and molarity. Less commonly used descriptions include normality and molality. A quantity of solute is measured out, mixed with solvent, and the volume is brought to some final quantity after the solute is completely dissolved.

That is, solutions are typically prepared volumetrically. Because solutes add volume to a quantity of solvent, this method of preparation of solutions is necessary to ensure that an exact desired concentration is obtained. If we partially fill an airtight syringe with air, the syringe contains a specific amount of air at constant temperature, say 25 °C. This example of the effect of volume on the pressure of a given amount of a confined gas is true in general.

Decreasing the volume of a contained gas will increase its pressure, and increasing its volume will decrease its pressure. In fact, if the volume increases by a certain factor, the pressure decreases by the same factor, and vice versa. Volume-pressure data for an air sample at room temperature are graphed in Figure 9.13. The line stops at 111 K because methane liquefies at this temperature; when extrapolated, it intersects the graph's origin, representing a temperature of absolute zero. The ideal gas equation contains five terms, the gas constant R and the variable properties P, V, n, and T. Specifying any four of these terms will permit use of the ideal gas law to calculate the fifth term as demonstrated in the following example exercises.

Volume-pressure data for an air sample at room temperature are graphed in Figure 5. Pour the tinted water into the 500-mL volumetric flask, using a funnel if needed. Pour the tinted ethyl alcohol into the 500-mL volumetric flask, using a funnel if needed. Note the level of the liquid in the 500-mL volumetric flask. (It should be very close to the 500-mL volume line.) Place a stopper or cap on the 500-mL volumetric flask and invert the flask several times. Remove the stopper or cap to prevent a vacuum from occurring inside flask.

Replace the cover and continue mixing the solution by inverting the flask until a green color is consistent throughout the solution. Allow the solution to settle, then observe the volume of liquid. (It should be far below the 500-mL volume line.) Mass the 500-mL volumetric flask containing the water-alcohol solution. Determine how much volume was lost in the creation of this solution. Using a disposable pipet, add water from a graduated cylinder to the volumetric flask containing the water-alcohol solution. Subtract this volume from 500 mL to obtain the final volume.

Ions with large hydrophobic surfaces such as tertaalkylammonium ions in effect increase hydrogen bonding in the adjacent water structure, which entails a further contribution, V∞caged. If this effect prevails, the ion is a structure-making ion, in contrast to the structure-breaking ions with a large V∞disorder term. Similarly, the appropriate value of heat capacity to use in a given process depends on whether the process produces a change in volume. The heat capacity is a function of the amount of heat added to a system.

In the case of a constant-volume process, all the heat affects the internal energy of the system (i.e., there is no pV-work, and all the heat affects the temperature). 1 mole of an ideal gas is compressed isothermally from volume of 20 L to 8 L against constant external pressure, when pressure volume work obtained is 44.9 L atm. This is an expression for pressure volume work done in terms of pressure and volume in the isothermal expansion of an ideal gas against constant external pressure. The volume units must be the same for both volumes in this equation. In general, M1 usually refers to as the initial molarity of the solution.

M2 refers to the final concentration of the solution and V2 is the final total volume of the solution. Imagine filling a rigid container attached to a pressure gauge with gas and then sealing the container so that no gas may escape. If the container is cooled, the gas inside likewise gets colder and its pressure is observed to decrease.

Density Of Air G Cm3 At Room Temperature

The relationship between the amounts of products and reactants in a chemical reaction can be expressed in units of moles or masses of pure substances, of volumes of solutions, or of volumes of gaseous substances. The ideal gas law can be used to calculate the volume of gaseous products or reactants as needed. A gas collected in such a way is not pure, however, but contains a significant amount of water vapor.

The measured pressure must therefore be corrected for the vapor pressure of water, which depends strongly on the temperature. Liquefaction can be viewed as an extreme deviation from ideal gas behavior. It occurs when the molecules of a gas are cooled to the point where they no longer possess sufficient kinetic energy to overcome intermolecular attractive forces. The precise combination of temperature and pressure needed to liquefy a gas depends strongly on its molar mass and structure, with heavier and more complex molecules usually liquefying at higher temperatures.

In general, substances with large van der Waals a coefficients are relatively easy to liquefy because large a coefficients indicate relatively strong intermolecular attractive interactions. Conversely, small molecules with only light elements have small a coefficients, indicating weak intermolecular interactions, and they are relatively difficult to liquefy. Gas liquefaction is used on a massive scale to separate O2, N2, Ar, Ne, Kr, and Xe. After a sample of air is liquefied, the mixture is warmed, and the gases are separated according to their boiling points. In Chapter 11 "Liquids", we will consider in more detail the nature of the intermolecular forces that allow gases to liquefy.

The behavior of ideal gases is explained by the kinetic molecular theory of gases. Molecular motion, which leads to collisions between molecules and the container walls, explains pressure, and the large intermolecular distances in gases explain their high compressibility. Although all gases have the same average kinetic energy at a given temperature, they do not all possess the same root mean square speed . The actual values of speed and kinetic energy are not the same for all particles of a gas but are given by a Boltzmann distribution, in which some molecules have higher or lower speeds than average. Diffusion is the gradual mixing of gases to form a sample of uniform composition even in the absence of mechanical agitation. In contrast, effusion is the escape of a gas from a container through a tiny opening into an evacuated space.

The rate of effusion of a gas is inversely proportional to the square root of its molar mass (Graham's law), a relationship that closely approximates the rate of diffusion. As a result, light gases tend to diffuse and effuse much more rapidly than heavier gases. The mean free path of a molecule is the average distance it travels between collisions. Eventually, these individual laws were combined into a single equation—the ideal gas law—that relates gas quantities for gases and is quite accurate for low pressures and moderate temperatures.

We will consider the key developments in individual relationships , then put them together in the ideal gas law. For a better understanding of how temperature and pressure influence air density, let's focus on a case of dry air. It contains mostly molecules of nitrogen and oxygen that are moving around at incredible speeds. Use our particles velocity calculator to see how fast they can move! For example, the average speed of a nitrogen molecule with a mass of 14 u (u - unified atomic mass unit) at room temperature is about 670 m/s - two times faster than the speed of sound!

Moreover, at higher temperatures, gas molecules further accelerate. As a result, they push harder against their surroundings, expanding the volume of the gas . And the higher the volume with the same amount of particles, the lower the density. Therefore, air's density decreases as the air is heated. We will now focus on macroscopic properties—the behavior of aggregates with large numbers of atoms, ions, or molecules.

An understanding of macroscopic properties is central to an understanding of chemistry. Why, for example, are many substances gases under normal pressures and temperatures (1.0 atm, 25°C), whereas others are liquids or solids? Of gases is the condensation of gases into a liquid form, which is neither anticipated nor explained by the kinetic molecular theory of gases. Both the theory and the ideal gas law predict that gases compressed to very high pressures and cooled to very low temperatures should still behave like gases, albeit cold, dense ones.

As gases are compressed and cooled, however, they invariably condense to form liquids, although very low temperatures are needed to liquefy light elements such as helium (for He, 4.2 K at 1 atm pressure). For an ideal gas, a plot of PV/nRT versus P gives a horizontal line with an intercept of 1 on the PV/nRT axis. Only at relatively low pressures do real gases approximate ideal gas behavior (part in Figure 10.21 "Real Gases Do Not Obey the Ideal Gas Law, Especially at High Pressures"). Real gases also approach ideal gas behavior more closely at higher temperatures, as shown in Figure 10.22 "The Effect of Temperature on the Behavior of Real Gases" for N2.

Density Of Air G Cm3 Why do real gases behave so differently from ideal gases at high pressures and low temperatures? Under these conditions, the two basic assumptions behind the ideal gas law—namely, that gas molecules have negligible volume and that intermolecular interactions are negligible—are no longer valid. At constant temperature, the kinetic energy of the molecules of a gas and hence the rms speed remain unchanged. If a given gas sample is allowed to occupy a larger volume, then the speed of the molecules does not change, but the density of the gas decreases, and the average distance between the molecules increases.

Hence the molecules must, on average, travel farther between collisions. They therefore collide with one another and with the walls of their containers less often, leading to a decrease in pressure. Air density equations Air contains a mixture of dry air and water vapor.

The amount of water vapor is a function of the relative humidity; it is also related to the dew point temperature of the air. If you need to calculate the density of dry air, you can apply the ideal gas law. This law expresses density as a function of temperature and pressure.

Like all gas laws, it is an approximation where real gases are concerned but is very good at low pressures and temperatures. Increasing temperature and pressure adds error to the calculation. Note that every molecule listed is heavier that or equal to 18 u.

Now, let's add some water vapor molecules to the gas with the total atomic weight of 18 u (H₂O - two atoms of hydrogen 1 u and one oxygen 16 u). According to Avogadro's law, the total number of molecules remains the same in the container under the same conditions . It means that water vapor molecules have to replace nitrogen, oxygen or argon.

Because molecules of H₂O are lighter than the other gases, the total mass of the gas decreases, decreasing the density of the air too. For Equation 10.27 to be valid, the identity of the particles present cannot have an effect. Thus an ideal gas must be one whose properties are not affected by either the size of the particles or their intermolecular interactions because both will vary from one gas to another.

The calculation of total and partial pressures for mixtures of gases is illustrated in Example 11. An online air density calculator such as the one by Engineering Toolbox let you calculate theoretical values for air density at given temperatures and pressures. The website also provides an air density table of values at different temperatures and pressures. These graphs show how density and specific weight decrease at higher values of temperature and pressure. The density of air is usually denoted by the Greek letter ρ, and it measures the mass of air per unit volume (e.g. g / m3).

Dry air mostly consists of nitrogen (~78 %) and oxygen (~21 %). The remaining 1 % contains many different gases, among others, argon, carbon dioxide, neon or helium. However, the air will cease to be dry air when water vapor appears.

Moreover, all molecules are attracted to one another by a combination of forces. These forces become particularly important for gases at low temperatures and high pressures, where intermolecular distances are shorter. Attractions between molecules reduce the number of collisions with the container wall, an effect that becomes more pronounced as the number of attractive interactions increases. At very high pressures, the effect of nonzero molecular volume predominates. The competition between these effects is responsible for the minimum observed in the PV/nRT versus P plot for many gases. A common use of Equation 10.23 is to determine the molar mass of an unknown gas by measuring its density at a known temperature and pressure.

This method is particularly useful in identifying a gas that has been produced in a reaction, and it is not difficult to carry out. A flask or glass bulb of known volume is carefully dried, evacuated, sealed, and weighed empty. It is then filled with a sample of a gas at a known temperature and pressure and reweighed. The difference in mass between the two readings is the mass of the gas. The volume of the flask is usually determined by weighing the flask when empty and when filled with a liquid of known density such as water. The use of density measurements to calculate molar masses is illustrated in Example 10.

Is defined as a hypothetical gaseous substance whose behavior is independent of attractive and repulsive forces and can be completely described by the ideal gas law. In reality, there is no such thing as an ideal gas, but an ideal gas is a useful conceptual model that allows us to understand how gases respond to changing conditions. As we shall see, under many conditions, most real gases exhibit behavior that closely approximates that of an ideal gas. The ideal gas law can therefore be used to predict the behavior of real gases under most conditions.

As you will learn in Section 10.8 "The Behavior of Real Gases", the ideal gas law does not work well at very low temperatures or very high pressures, where deviations from ideal behavior are most commonly observed. Figure 10.2 "Elements That Occur Naturally as Gases, Liquids, and Solids at 25°C and 1 atm" shows the locations in the periodic table of those elements that are commonly found in the gaseous, liquid, and solid states. Except for hydrogen, the elements that occur naturally as gases are on the right side of the periodic table.

Of these, all the noble gases are monatomic gases, whereas the other gaseous elements are diatomic molecules . Oxygen can also form a second allotrope, the highly reactive triatomic molecule ozone , which is also a gas. In contrast, bromine and mercury are liquids under normal conditions (25°C and 1.0 atm, commonly referred to as "room temperature and pressure"). Gallium , which melts at only 29.76°C, can be converted to a liquid simply by holding a container of it in your hand or keeping it in a non-air-conditioned room on a hot summer day.

The rest of the elements are all solids under normal conditions. In contrast, the macroscopic properties of a substance depend strongly on its physical state, which is determined by intermolecular forces and conditions such as temperature and pressure. This section gives you a first insight into the basics of density measurement. You will learn that density is a temperature and pressure-dependent substance property which is often specified with the unit kg/m3 or lb/ft3.

The density value is required for determining concentration, average molecular weight and content. For finding the density of gases, it must be noted that this density depends on the respective pressure. The pressure at any point in a static fluid depends only on the depth at that point. As discussed, pressure in a fluid near Earth varies with depth due to the weight of fluid above a particular level. In the above examples, we assumed density to be constant and the average density of the fluid to be a good representation of the density. This is a reasonable approximation for liquids like water, where large forces are required to compress the liquid or change the volume.

In a swimming pool, for example, the density is approximately constant, and the water at the bottom is compressed very little by the weight of the water on top. Traveling up in the atmosphere is quite a different situation, however. The density of the air begins to change significantly just a short distance above Earth's surface. In the following example, the mass is found directly by weighing, but the volume is found indirectly through length measurements. The density of a substance is the ratio of the mass of a sample of the substance to its volume.

The SI unit for density is the kilogram per cubic meter (kg/m3). For many situations, however, this as an inconvenient unit, and we often use grams per cubic centimeter (g/cm3) for the densities of solids and liquids, and grams per liter (g/L) for gases. Common units for density include g/mL, g/cm3, g/L, or kg/L. Although there are exceptions, most liquids and solids have densities that range from about 0.7 g/mL to 19 g/mL . For any ideal gas, at a given temperature and pressure, the number of molecules is constant for a particular volume (see Avogadro's Law).

So when water molecules are added to a given volume of air, the dry air molecules must decrease by the same number, to keep the pressure or temperature from increasing. Although the two terms often are used interchangeably, there is a technical difference between specific gravity and density. Density is defined as the mass per unit volume of a substance. When the specific gravity is defined based on water at 4°C, then the specific gravity is equal to the density of the liquid. However, if the specific gravity is expressed at different temperatures, it will no longer be equal to the density.

Although there is a difference between specific gravity and density, for the most part the values are similar enough to be used interchangeably in most situations. A and b are empirical constants that are different for each gas. The values of a and b are listed in Table 10.5 "van der Waals Constants for Some Common Gases" for several common gases. The pressure term—P+ (an2/V2)—corrects for intermolecular attractive forces that tend to reduce the pressure from that predicted by the ideal gas law. The volume term—V−nb—corrects for the volume occupied by the gaseous molecules.

Because the molecules of an ideal gas are assumed to have zero volume, the volume available to them for motion is always the same as the volume of the container. In contrast, the molecules of a real gas have small but measurable volumes. As a result, the volume occupied by the molecules becomes significant compared with the volume of the container.

Consequently, the total volume occupied by the gas is greater than the volume predicted by the ideal gas law. Thus at very high pressures, the experimentally measured value of PV/nRT is greater than the value predicted by the ideal gas law. The ideal gas law allows us to calculate the value of the fourth variable for a gaseous sample if we know the values of any three of the four variables . It also allows us to predict the final state of a sample of a gas (i.e., its final temperature, pressure, volume, and amount) following any changes in conditions if the parameters are specified for an initial state. Some applications are illustrated in the following examples. The approach used throughout is always to start with the same equation—the ideal gas law—and then determine which quantities are given and which need to be calculated.